10 multiple choice calculus - CA Essay Writers

The graph of f ‘(x) is continuous, positive, and has a relative maximum at x = 0. Which of the following statements must be true? (5 points)

	The graph of f is always concave down.
	The graph of f is always increasing.
	The graph of f has a relative maximum at x = 0.
	The graph of f has a relative minimum at x = 0.

Below is the graph of f ‘(x), the derivative of f(x), and has x-intercepts at x = -3, x = 1, and x = 2 and a relative maximum at x = -1.5 and a relative minimum at x = 1.5. Which of the following statement is false?

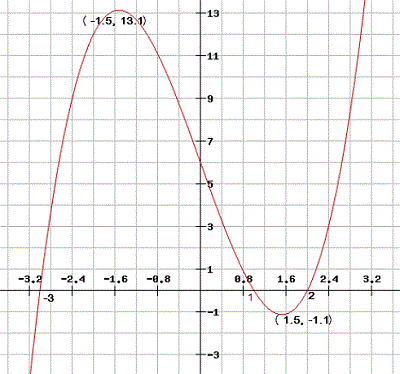Graph of a function that increases from negative infinity to x equals negative 1.5, decreases from x equals negative 1.5 to x equals 1.5, crossing the y axis at y equals 6, and increases from x equals 1.5 to positive infinity with x intercepts at x equals negative 3, 1 and 2. (5 points)

	f is concave up from x = -1.5 to x = 1.5.
	f has an inflection point at x = 1.5.
	f has a relative minimum at x = 2.
	All of these are false.

The graph of y = f ‘(x), the derivative of f(x), is shown below. List the intervals where the graph of f is concave down.

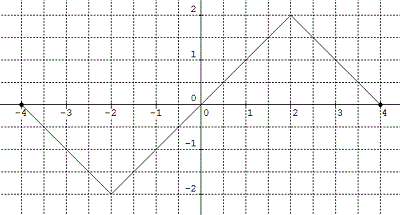Graph consists of 3 line segments from x equals negative 4 to x equals 4. Graph is decreasing from x equals negative 4 to x equals negative 2, increases from x equals negative 2 to x equals 2 and decreases from x equals 2 to x equals 4. There are x intercepts at x equals negative 4, 0 and 4. (5 points)

	(-4, -2) U (2, 4)
	(-2, 2)
	(-4, 0)
	(0, 4)

Which of the following functions grows the fastest as x grows without bound? (5 points)

	f(x) = e^x
	g(x) = e^cosx
	h(x) =
	They all grow at the same rate.

Compare the growth rate of the functions f(x) = x³ + 1 and g(x) = x² . (5 points)

	f(x) grows faster than g(x).
	g(x) grows faster than f(x).
	f(x) and g(x) grow at the same rate.
	It cannot be determined.

f is a function that is differentiable for all reals. The value of f ‘(x) is given for several values of x in the table below.

x	-8	-3	0	3	8
f ‘(x)	-4	-2	0	4	5

If f ‘(x) is always increasing, which statement about f(x) must be true? (5 points)

	f(x) passes through the origin.
	f(x) is concave downwards for all x.
	f(x) has a relative minimum at x = 0.
	f(x) has a point of inflection at x = 0.

f is a differentiable function on the interval [0, 1] and g(x) = f(2x). The table below gives values of f ‘(x). What is the value of g ‘(0.1)? (5 points)

x	0.1	0.2	0.3	0.4	0.5
f ‘(x)	1	2	3	-4	5

	1
	2
	4
	Cannot be determined

Use the graph of f(t) = 2t + 2 on the interval [-1, 4] to write the function F(x), where 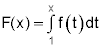f of x equals the integral from 1 to x of f of t dt . (5 points)

	F(x) = x² + 3x
	F(x) = x² + 2x – 12
	F(x) = x² + 2x – 3
	F(x) = x² + 4x – 8

The velocity of a particle moving along the x-axis is v(t) = t² + 2t + 1, with t measured in minutes and v(t) measured in feet per minute. To the nearest foot find the total distance travelled by the particle from t = 0 to t = 2 minutes. (5 points)

10.

Find the range of the function 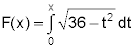f of x equals the integral from 0 to x of the square root of the quantity 36 minus t squared dt . (5 points)

	[-6, 6]
	[0, 6]
	[0, 9Ï€]
	[0, 18Ï€]